Let's use the variable x to represent the jet speed and y to represent the jetstream speed.
If the jet travels 5008 miles in 8 hours against the jetstream (that is, a relative speed of x - y), we can write the equation:
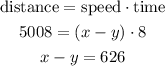
Then, if the jet travels 5968 miles in 8 hours with the jetstream (relative speed of x + y), so we have:
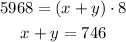
Adding the equations we found, we can find the value of x:
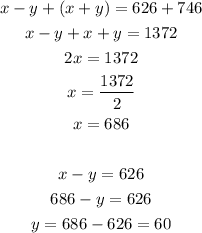
So the jet speed is 686 mph and the jetstream speed is 60 mph.