Question

a scientist has two solutions, which she has labeled Solution A & Solution B. each contains salt. she know solution A is 40% salt and solution B is 55% salt. she wants to obtain 180 ounces of a mixture that is 45% salt. how many ounces of each solution should she use?

Answer 1

Let the number of ounces of solution A be

`=x`

Let the number of ounces of solution B be

`=y`

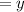
The total number of ounces of the mixture is

`=180`

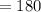
Therefore,

The equation to represent this will be

`x+y=180\ldots\ldots(1)`

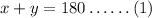
The percentage of salt in solution A is

`\begin{gathered} =40\% \\ =(40)/(100)=0.4 \end{gathered}`

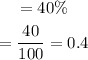
The percentage of salt in solution B is

`\begin{gathered} =55\% \\ =(55)/(100)=0.55 \end{gathered}`

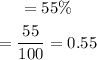
The percentage of salt in the mixture is

`\begin{gathered} =45\% \\ =(45)/(100)=0.45 \end{gathered}`

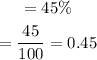
Therefore,

The equation to represent the percentage of salt is given below as

`\begin{gathered} 0.4x+0.55y=0.45*180 \\ 0.4x+0.55y=81 \\ \text{ multiply through by 100} \\ 40x+55y=8100\ldots\text{.}(2) \end{gathered}`

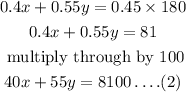
Step 1:

From equation (1) make x the subject of the formula

`\begin{gathered} x+y=180 \\ x=180-y\ldots\text{.}\mathrm{}(3) \end{gathered}`

Step 2:

Substitute equation (3) in equation (2)

`\begin{gathered} 40x+55y=8100 \\ 40(180-y)+55y=8100 \\ 7200-40y+55y=8100 \\ 15y=8100-7200 \\ 15y=900 \\ \text{divide both sides by 15} \\ (15y)/(15)=(900)/(15) \\ y=60 \end{gathered}`

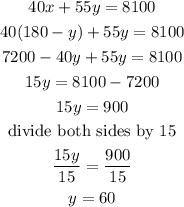
Step 3:

Substitute y=60 in equation (3)

`\begin{gathered} x=180-y \\ x=180-60 \\ x=120 \end{gathered}`

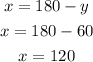
Hence,

The number of ounces of solution A = 120

The number of ounces of solution B = 60