Question

Jake is helping Fin push a box at a constant velocity up an incline that makes an angle of 30.0° above the horizontal by applying a 94.0N force in line with the incline over 2.30m. If the coefficient of kinetic friction between the box and the incline is 0.280, a) what is the work done by the pushing force, in Joules? b) What is the mass of the box, in kilograms?

Answer 1

Given data

The angle of inclination of the plane is theta = 30 degree

The applied force in the inclined plane is F = 94 N

The distance moved in the inclined plane is d = 2.30 m

The coefficient of kinetic friction is u_k = 0.280

The free-body diagram of the above configuration is shown below:

Here, the normal reaction force on the box is N, the acceleration due to gravity is denoted as g, the friction force on the box is F_f, and the mass of the box is denoted as m.

(a)

The expression for the work done by the pushing force is given as:

`W=Fd`

Substitute the value in the above equation.

`\begin{gathered} W=94\text{ N}*2.30\text{ m} \\ W=216.2\text{ J} \end{gathered}`

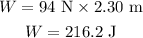
Thus, the work done by the pushing force is 216.2 J.

(b)

The box is moving at the constant velocity, therefore, the pushing force will be equal to the frictional force and the component of the gravitational force in the inclined plane.

`\begin{gathered} F=F_f+mg\sin \theta \\ F=\mu_kN+mg\sin \theta \end{gathered}`

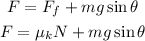
The expression for the normal reaction force is given as:

`N=mg\cos \theta`

The expression for the mass of the box is given as:

`\begin{gathered} F=\mu_k* mg\cos \theta+mg\sin \theta \\ m=(F)/(\mu_kg\cos \theta+g\sin \theta) \end{gathered}`

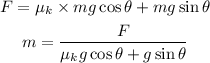
Substitute the value in the above equation.

`\begin{gathered} m=\frac{94\text{ N}}{0.28*9.8m/s^2*\cos 30^o+9.8m/s^2*\sin 30^0} \\ m=12.9\text{ kg} \end{gathered}`

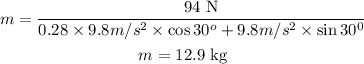
Thus, the mass of the box is 12.9 kg.