Question

At the top of a 170 m tall building, a 4 kg ball is dropped from rest. The ball strikes the ground with a speed of 30 m/s. How much energy is lost to air friction?

Answer 1

We are given that an object is dropped from rest from the top of a building and we are asked to determine the energy lost due to friction when it hits the bottom. To do that we will use an energy balance between the top of the building and the point where the object hits the floor. At the top, we have potential energy and at the bottom, we have kinetic energy. The potential energy turns into kinetic energy and the energy lost due to friction therefore, the balance of energy is:

`E_p-E_f=E_k`

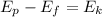
Where:

`\begin{gathered} E_p=\text{ potential energy} \\ E_f=\text{ energy lost} \\ E_k=\text{ kinetic energy} \end{gathered}`

Now we solve for the energy lost first by subtracting the potential energy from both sides:

`-E_f=E_k-E_p`

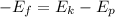
Now we multiply both sides by -1:

`E_f=E_p-E_k`

The potential energy is given by:

`E_p=\text{mgh}`

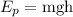
Where:

`\begin{gathered} m=\text{ mass} \\ g=\text{ acceleration of gravity} \\ h=\text{ height} \end{gathered}`

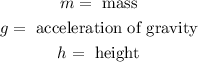
The kinetic energy is given by:

`E_k=(1)/(2)mv^2`

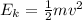
Where:

`v=\text{ velocity}`

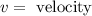
Substituting in the energy balance:

`E_f=\text{mgh-}(1)/(2)mv^2`

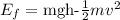
Now we substitute the values:

Solving the operations:

`E_f=4864J`

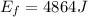
Therefore, the energy lost due to friction is 4864 Joules.