Solution:
Given:
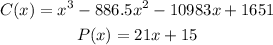
The revenue is given by;
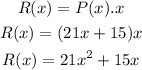
Hence, the profit is the difference between the revenue and the cost.
Thus,
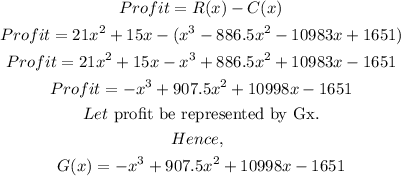
To get the maximum profit, we differentiate the profit function and equate it to zero.
Hence,
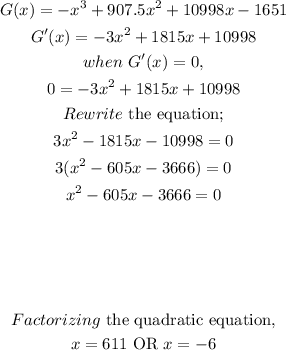
To get the maximum production level, we differentiate further.

Therefore, the production level that will maximize profit is x = 611.