The rectangular prism has 6 rectangular faces, to determine the surface area of the prism, you have to calculate the area of all faces and then add them.
The opposite sides of the prism are equal, so you have three pairs of rectangles whose areas you have to determine:
-(1) Top and bottom faces of the prism.
These sides lengths are the length and width of the prism, their area is the product between both lengths, and, since there are two faces, you have to multiply the area by 2:
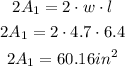
-(2)Back and front
These sides lengths are the length and height of the prism, and their area combined is twice the product of the length and the height:

-(3)Left and right faces
These sides lengths are the width and height of the prism. Their area combined is twice the product between them:
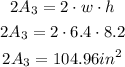
Once all areas are calculated, you have to add them to determine the surface area (SA) of the rectangular prism:

The surface area of the prism is 242.2in²