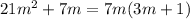Question

Use HCF method , DOTS method or Trinomial method based on the equation given. Numer 5

Answer 1

We are given the following expression:

`21m^2+7m`

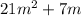
To factor the expression we will use the HCF method. We will determine the Greatest Common Multiplier of the terms of the expression. First, we'll determine the GCM of the coefficients. The factors of the coefficients are:

`\text{factors 21 = }1,3,7,21`
`\text{Factors 7 = }1,7`

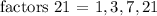
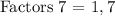
Therefore, the greatest common multiplier is 7.

Now, the GCM of the variables is the variables that repeat in each term elevated to the minimum coefficient. The variable that repeats is "m" and the least exponent is 1, therefore, the GCM is:

`7m`

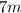
Therefore, the factorization is:

`21m^2+7m=7m(3m+1)`