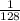Given:
A single bacterium is placed in a bottle at 11:00 am.
It grows and at 11:01 divides into two bacteria.
These two bacteria each grow and at 11:02 divide into four bacteria.
These four bacteria each grow and at 11:03 divide into eight bacteria.
The bottle is full at 12:00.
To find:
The number of bacteria are in the bottle at 11:53 and the fraction of the bottle is full at that time.
Step-by-step explanation:
After 1 min, the number of bacterias is,
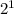
After 2 min, the number of bacterias is,
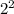
After 3 min, the number of bacterias is,
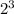
In general,
After t min, the number of bacteria is,
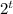
If the bottle is full at 12:00 that means after 60 mins, then the number of bacteria is,
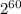
Therefore, the number of bacteria at 11:53 is,
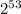
Then the fraction of the bottle is full at 11:53 is,

Final answer:
• The number of bacteria at 11:53 is,
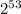
• The fraction of the bottle is full at 11:53 is,