Test each of the options to get those that are not a trigonometry identites
For option A

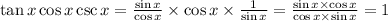
OPTION A IS A TRIGONOMETRY IDENTITY
For option B
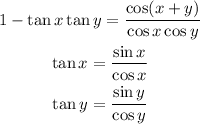
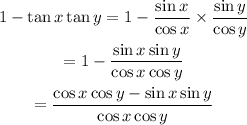
Therefore,
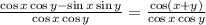
Multiply through by the common base
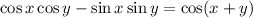
The above expression is a trigonometry identity, so it is true.
OPTION B IS A TRIGONOMETRY IDENTITY
Checking for option C

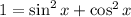
The above is a triogonometry identity
OPTION C IS A TRIGONOMETRY IDENTITY
Checking for option D
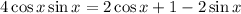
The above is not a trigonometry identity
OPTION D IS NOT A TRIGONOMETRY IDENTITY
Hence, the option that is not a trigonometry identity is OPTION D