Question

Solve the equation. - 4(r + 6) = -54

Answer 1

- 4(r + 6) = -54​

expand

-4r - 24 = -54

add 24 to both sides

-4r = -54 + 24

-4r = -30

Divide both sides by -4

r = -30/-4

r = 7.5

Answer 2

`\boxed{\sf{r=(15)/(2)=7.5 }}`

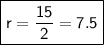
Explanation:

Use the distributive property.

a(b+c)=ab+ac

-4(r+6)=-54

Isolate the term of r from one side of the equation.

You have to divide by -4 from both sides.

`\sf{(-4\left(r+6\right))/(-4)=(-54)/(-4)}`

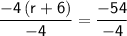
Solve.

-54/-4=27/2

r+6=27/2

Subtract by 6 from both sides.

r+6-6=27/2-6

Solve.

`\sf{-(6* \:2)/(2)+(27)/(2)}}`

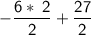
-6*2+27/2

Remember to solve this problem, first thing you do is solve with order of operations.

PEMDAS stands for:

• Parentheses
• Exponents
• Multiply
• Divide
• Add
• Subtract

Do multiply first.

-6*2=-12

-12+27

Then add or subtract the numbers from left to right.

-12+27=15

r=15/2

Divide is another option.

15/2=7.5

`\rightarrow \boxed{\sf{r=(15)/(2)=7.5}}`

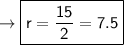
Therefore, the correct answer is r=15/2=7.5.

I hope this helps, let me know if you have any questions.