Question

This is what I have so far. I do not know what to do next.

Answer 1

We are given the following two equations

`\begin{gathered} 3x+y=-5\quad eq.1 \\ 6x+2y=10\quad eq.2 \end{gathered}`

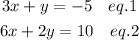
Let us solve the system of equations using the substitution method.

Separate out one of the variables in any of the two equations

Let us separate out x from eq.1

`\begin{gathered} 3x+y=-5 \\ 3x=-5-5 \\ x=(-y-5)/(3) \end{gathered}`

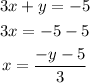
Now, substitute this value of x into eq.2

`\begin{gathered} 6x+2y=10\quad eq.2 \\ 6((-y-5)/(3))_{}+2y=10 \\ 2(-y-5)_{}+2y=10 \\ -2y-10+2y=10 \\ -10=10 \end{gathered}`

The variable y also got canceled and we are left with something that cannot be true. (-10 cannot be equal to 10)

This means that the system of equations has infinitely many solutions.