Question

Out of 479 applicants for a job. 249 have over 10 years of experience and 62 have over 10 years of experience and have a graduate degree.Step 1 of 2: What is the probability that a randomly chosen applicant has a graduate degree, given that they have over 10 years of experience? Enter a fraction orround your answer to 4 decimal places, if necessary.

Answer 1

Given:

Total number of applicants = 479

Number who have over 10 years experience = 249

Number who have over 10 years experience and a graduate degree = 62

Let's find the probability that a randomly chosen applicant has a graduate degree, given that they have over 10 years experience.

To find the probability, we have:

`P=\frac{P(graduate\text{ and 10 years experience\rparen}}{P(10\text{ years experience\rparen}}`

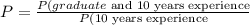
Where:

`\begin{gathered} P(graduate\text{ and 10 years experience\rparen = }(62)/(479) \\ \\ \\ P(0ver\text{ 10 years experience\rparen = }(249)/(479) \end{gathered}`

Thus, we have:

`\begin{gathered} P=((62)/(479))/((249)/(279)) \\ \\ P=(62)/(479)*(479)/(249) \\ \\ P=(62)/(249) \\ \\ P=0.2490 \end{gathered}`

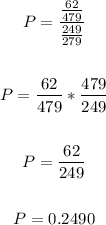
Therefore, the probability is 0.2490

ANSWER:

0.2490