Question

Find ST R. 12.5 x + 5 V 7.5 S X-5 T O A 25 B. 20 O C. 10 OD. 15

Answer 1

`ST\text{ = 15}`

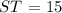
Here, we want to calculate the value of ST

To do this, we need to check for similar triangles

As we can see; we have similar triangles and thus, will use the bisector theorem

We have this as;

`\begin{gathered} (x-5)/(x+5)\text{ = }(7.5)/(12.5) \\ \\ 12.5(x-5)\text{ = 7.5(x+5)} \\ 12.5x\text{ - 62.5 = 7.5x + 37.5} \\ 12.5x\text{ - 7.5x = 37.5 + 62.5} \\ 5x\text{ = 100} \\ x\text{ = }(100)/(5) \\ \text{ x = 20} \end{gathered}`

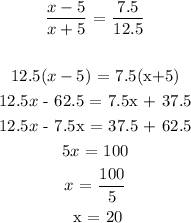
But ST is x-5

Simply substitute the value of x in the expression

We have this as; 20-5 = 15