Question

A uniform board 6.2 m long with a mass of 65 kg is attached to a wall with a pivot. A wooden crate sits in the board 2 m from the left edge. A rope with a tension of 800 N that makes an angle of 45 degrees with the horizontal is attached 0.3 m from the left end of the beam and connects the beam to the same wall as the pivot to keep the system balanced. What is the mass of the wood crate?

Answer 1

92.09 kg.

Explanation:

Mass board (M) = 65 kg

Length board (L) = 6.2 m

Left edge wooden (l) = -2 m

Tension (T) = 800 N

There are a total of 3 forces acting on the board, they are the following:

`\begin{gathered} W_(board)=M\cdot g=65\cdot9.8=637\text{ N} \\ \\ W_(wooden)=mg=m(9.8)=9.8m\text{ N} \\ \\ T_y=T\cdot\sin\theta=800\cdot\sin45\degree=565.7\text{ N} \end{gathered}`

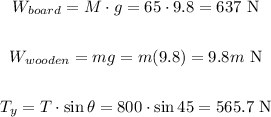
Since the board is balanced, the net torque acting up will be equal to the net torque acting down.

So we can establish the following balance:

`\begin{gathered} \tau_(tension)=\tau_(board)+\tau_(wooden) \\ \\ T_y\cdot d=W_(board)\cdot(L)/(2)+W_(wooden)\cdot l \\ \\ \text{ We replacing:} \\ \\ 565.7\cdot0.3=637\cdot(6.2)/(2)+9.8m\cdot-2 \\ \\ 169.71=1974.7-19.6m \\ \\ m=(1974.7-169.71)/(19.6) \\ \\ m=92.09\text{ kg} \end{gathered}`

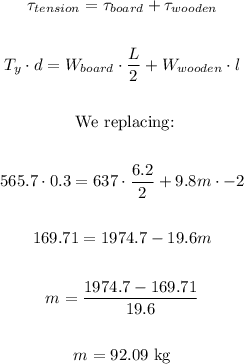
The mass of the wooden crate is 92.09 kg.