Question

a store pays $10 for a bracelet and the markup is 115%. A customer will also pay 5 and a half sale tax . what will be the total cost to the nearest cent ?

Answer 1

$22.68

Explanation:

Given:

Bracelet = $10

Markup percentage = 115% = 115/100 = 1.15

The markup amount can be calculated as follows:

`\begin{gathered} \text{ markup amount = cost price }\cdot\text{ markup percentage} \\ \text{ replacing} \\ \text{markup amount }=10\cdot1.15=11.5 \end{gathered}`

Therefore, the total price would be:

`t=10+11.5=21.5`

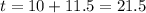
Now we apply sales tax for the price and calculate the price after tax, like this:

`\begin{gathered} t=21.5+21.5\cdot(5.5)/(100) \\ t=21.5+1.1825 \\ t=22.6825\cong22.68 \end{gathered}`

$22.68 is the total cost of the bracelet