Given the equation of a straight line

m is the slope and c is the intercept on the y axis
From the table in the question, the equation of the line can be obtained using two points form the equation of a straight line
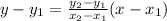
Pick two points on the table and substitute for the x values and y values
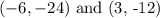

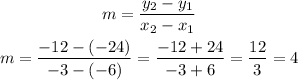
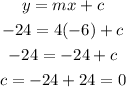
Hence, the equation of the table is y = 4x