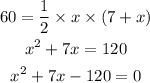Question

One leg of a right triangle is 7cm longer than the shorter leg, the hypotenuse is 17cm, and the area is 60 cm2. Recall the two formulas related to triangles are a2+b2=c2 and Area=1/2(base)(height).a. Sketch the triangle using x for the length of the shorter side, be sure to include all the given information provided in your sketch.b. Give the equation you would use to solve for the missing sides using the length of the hypotenuse...DO NOT SOLVE.c. give the equation you would use to solve for the missing sides using the area...DO NOT SOLVE.

Answer 1

Let the shorter leg be x cm;

then the other side is (7+x)cm;

the hypotenuse side is 17cm;

and the area of the right-angled triangle is 60 square centimeters.

(a)

(b) Recall the pythagorean theorem that the square of the longest side (hypotenuse) is equal to the sum of the squares of the two remaining sides.

`\begin{gathered} (7+x)^2+x^2=17^2 \\ 49+14x+x^2+x^2=289 \\ 2x^2+14x-240=0 \end{gathered}`

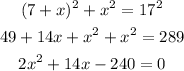
(c) The area of a triangle is given as;

`\begin{gathered} 60=(1)/(2)* x*(7+x) \\ x^2+7x=120 \\ x^2+7x-120=0 \end{gathered}`