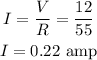ANSWER:
55Ω
I = 0.22 amp
Explanation:
We are divided into two parts, the first is to solve the parallel system, like this:
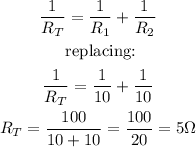
Then we have a series system, therefore, we solve like this:
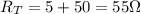
The first thing is to calculate the current, knowing that the voltage is equal to 12V, like this: