Register
What is the lowest common multiple of 28 & 6
112k views
3 votes
What is the lowest common multiple of 28 & 6

User FLC
by
6.2k points

1 Answer

5 votes

Given:

The objective is to find the lowest common multiples of 28 and 6.

Step-by-step explanation:

The common multiple can be calculated using division method as,

Now, the LCM can be calculated as,


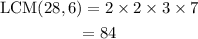
\begin{gathered} \text{LCM}(28,6)=2*2*3*7 \\ =84 \end{gathered}

Hence, the lowest common multiples of 28 and 6 are 84.

What is the lowest common multiple of 28 & 6-example-1
User Bimawa
by
5.8k points
Ask a Question
Welcome to QAmmunity.org, where you can ask questions and receive answers from other members of our community.

6.6m questions

8.8m answers

Other Questions

What is the least common denominator of the four fractions 20 7/10 20 3/4 18 9/10 20 18/25
What is 0.12 expressed as a fraction in simplest form
arlos has $690,000 he wants to save. If the FDIC insurance limit per depositor, per bank, is $250,000, which of these ways of distributing his money between three banks will guarantee that all of his money
What is distributive property ?
What equation results from completing the square and then factoring x^2+4x=12? A) (x+2)^2=10 B) (x+2)^2=14 C) (x+2)^2=16 D) (x+2)^2=8
Link Copied!