Question

A near function contains the three ordered pairs shown in the table. 1 5 6 7 7 Create an equation for a different linear function that has twice the rate of change and the same initial value as the one represented by the table. Do not include spaces in your response. Remember fractions such as are typed as 1/4

Answer 1

The ordered pairs are (1,4), (5,6) and (7,7) of the form (n,g)

The rate of change is the slope of the equation

`\begin{gathered} (g_2-g_1)/(n_2-n_1)=(6-4)/(5-1)=(2)/(4)=(1)/(2) \\ m=(1)/(2) \end{gathered}`

The equation of the linear function now becomes;

`\begin{gathered} m=(g-g_1)/(n-n_1) \\ (1)/(2)=(g-4)/(n-1) \\ 2(g-4)=n-1 \\ 2g-8=n-1 \\ 2g=n-1+8 \\ 2g=n+7 \\ \text{Divide all through by 2} \\ g=(1)/(2)n+(7)/(2) \\ g=(1)/(2)n+3.5 \end{gathered}`

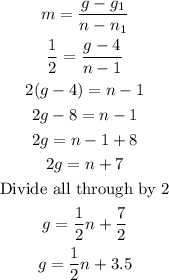
Hence the equation of the linear function is g = 0.5n + 3.5

Another equation for a function that has twice the rate of change and the same initial value becomes;

initial rate of change is 0.5

hence for this, the rate of change is 0.5 x 2

m = 1

the initial value is at n = 0,

so g = 0.5(0) + 3.5

g = 3.5

The new equation then becomes g = mn + C

g = 1n + 3.5

Hence the new equation that has twice the rate of change and the same initial value is g = n + 3.5