Answer
2.1 The mole of Ba(OH)₂ that react = 5.33 mol
2.2 The moles of H₂SO₄ that are left unreacted = 7.17 mol
Step-by-step explanation
Given:
Volume of Ba(OH)₂ = 15 cm³ = (15/1000) = 0.015 dm³
Concentration of Ba(OH)₂ = 0.08 mol/dm³
Volume of H₂SO₄ = 20 cm³ = (20/1000) = 0.020 dm³
Concentration of H₂SO₄ = 0.25 mol/dm³
Equation: Ba(OH)₂ (aq) + H₂SO₄ (aq) -----> BaSO₄ (s) + H₂O (l)
What to find:
2.1 The mole of Ba(OH)₂ that react.
2.2 The moles of H₂SO₄ that are left unreacted.
Step-by-step solution:
Step 1: Convert the given concentrations of the reactants to moles.
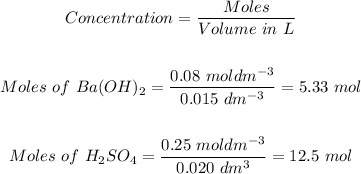
Step 2: 2.1 The mole of Ba(OH)₂ that react.
Comparing the moles of each of the reactants in step 1 with the mole ratio of the reactants in the given reaction, it can be concluded that 5.33 moles of Ba(OH)₂ react.
Hence, the mole of Ba(OH)₂ that react is 5.33 mol.
Step 3: The moles of H₂SO₄ that are left unreacted.
From the given balanced chemical equation for the reaction; the mole ratio of Ba(OH)₂ to H₂SO₄ is 1:1
Also, 5.33 moles of H₂SO₄ will react out of the 12.5 moles of H₂SO₄.
Therefore, the moles of H₂SO₄ that are left unreacted = (12.50 mol - 5.33 mol) = 7.17 mol
The moles of H₂SO₄ that are left unreacted would be 7.17 moles