Question

A loan of $12,651 was repaid at the end of 8 months What size repayment check (principal and interest) was written, if a 7.5% annual rate of interest was charged?The amount of the repayment check was $(Round to two decimal places)

Answer 1

We have a loan with a principal of $12,651.

It is paid in 8 monthly payments.

The annual rate of interest is 7.5%.

We then have to calculate the amount of each monthly payment.

This can be calculated using the annuity formula with subperiods.

The formula is:

`M=(P\cdot(r)/(m))/(1-(1+(r)/(m))^(n\cdot m))`

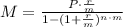
For this problem we have a principal P = 12,651, an interest rate r = 0.075, the subperiods are months so m = 12, and the number of payments is n*m = 8.

We can replace and solve as:

`\begin{gathered} M=(12651\cdot(0.075)/(12))/(1-(1+(0.075)/(12))^(-8)) \\ M=(12651\cdot0.00625)/(1-(1+0.00625)^(-8)) \\ M=(79.06875)/(1-(1.00625)^(-8)) \\ M\approx(79.06875)/(1-0.95138) \\ M\approx(79.06875)/(0.04862) \\ M\approx1626.26 \end{gathered}`

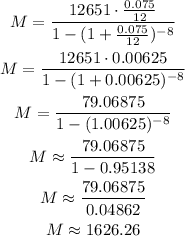
Answer: the monthly payment in each check was $1626.26.