Question

determine the following for g(x) = 3x+600 / x^2 - 90xwrote none if a graphical feature does not exist

Answer 1

We will have the following:

1st:

* Determinant:

`d=(-8)^2-4(2)(9)\Rightarrow d=-8`

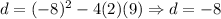
* #x-intecepts: GIven the determinant we can see that there are no x-intercepts.

2nd:

`g(x)=(3x+600)/(x^2-90x)`

*Verical Asymptotes:

`(3x-600)/(x^2-90x)=0\Rightarrow\begin{cases}x^2-90x=0\Rightarrow\end{cases}x(x-90)=0`
`\Rightarrow\begin{cases}x=0 \\ \\ x=90\end{cases}`

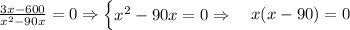
So, there are two vertical asymptotes at x = 0 & x = 90.

*Horizontal Asymptotes:

We can see that the degree of the denominator is greater than that of the numerator so, there is a horizontal asymptote at y = 0.

*x-Intercept:

Since there is a horizontal asymptote at y = 0 and the function becomes undefined when x = 0, there is no x-intercept in this function. [Being very technical it could be stated that there are x-intercepts at (-∞, 0) & (∞, 0), but since these vales are undefined they are of little use]