The centripetal force is given by:
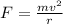
In this case we know the velocity, mass and radius but we need to use the proper units for the velocity, so let's make the conversion to m/s:
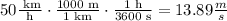
Plugging the values given and the value of the velocity in the correct units we have that:

Therefore the centripetal force acting on the car is 3062.41 N