Question

Watch help video-The function y f(x) is graphed below.What is the average rate of change of thefunction f(x) on the interval—7 < x < -3?100

Answer 1

The formula to calculate the average rate of change of a function f(x) over the interval:

`a\leq x\leq b`

is given to be:

`AROC=(f(b)-f(a))/(b-a)`

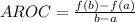
From the provided question, we have:

`\begin{gathered} a=-7 \\ b=-3 \end{gathered}`

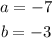
From the graph, we have:

`\begin{gathered} f(a)=-10 \\ f(b)=30 \end{gathered}`

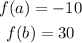
Therefore, the average rate of change is:

`\begin{gathered} AROC=(30-(-10))/(-3-(-7))=(30+10)/(-3+7)=(40)/(4) \\ AROC=10 \end{gathered}`

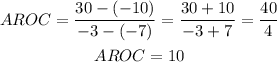
Therefore, the average rate of change is 10.