Question

Mrs, Singh purchased how many soft cover books?Mrs, Singh purchase how many hardcover books?

Answer 1

Step-by-step explanation

In the question, we are given that Mrs.Singh purchased 31 softcover books and hardcover books. Let softcover books and the hardcover book be x and y respectively.

Therefore;

`x+y=31----1`

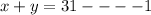
Also, each softcopy book costs $3.50 and hard copy books cost $14.50.

`3.50x+14.50y=240.50---2`

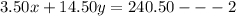
The next step is to solve the simultaneous equation.

`x=31-y------3`

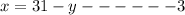
Substitute equation iii into ii

`\begin{gathered} 3.50(31-y)+14.50y=240.50 \\ 108.50-3.50y+14.50y=240.50 \\ 11y=240.50-108.50 \\ 11y=132 \\ (11y)/(11)=(132)/(11) \\ y=12 \end{gathered}`

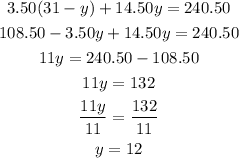
Next, we substitute the value of y in equation iii

`\begin{gathered} x=31-12 \\ x=19 \end{gathered}`

Therefore the answers are:

Soft cover books: 19 books

Hard coverbooks: 12 books