Question

the side opposite the 60 degrees angle measure's 10 cm. what is the measure of the hypotenuse? (draw and label the triangle before solving)

Answer 1

you

11.54 cm

Step-by-step explanation

Step 1

draw and label

we have a right triangle, then

Step 2

Let

`\begin{gathered} \text{angle}=\emptyset=60\text{ \degree} \\ \text{opposite side= 10 cm} \\ \text{hypotenuse = h} \end{gathered}`

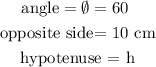
hence, we need a function that relates those values, it is sine function:

The sine function is a periodic function which is very important in trigonometry, it says for a rigth triangle, the sine of angle is the ratio of opposite side to hypotenuse: so

`\sin \emptyset=\frac{opposite\text{ side}}{\text{hypotenuse}}`

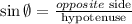
finally, replace and solve for h

`\begin{gathered} \sin \emptyset=\frac{opposite\text{ side}}{\text{hypotenuse}} \\ \sin \text{ 60=}(10)/(h) \\ h\cdot\sin 60=10 \\ h=\frac{10}{\sin \text{ 60}} \\ h=11.54 \end{gathered}`

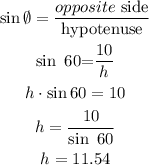
therefore, the hypotenuse is

11.54 cm

I hope this helps you