The general form of an equation is given by
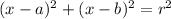
where (a,b) are the coordinates of the centre and r is the radius of the circle.
Now the coordinates of the centre are the midpoint of the points (2,6) and (8,-6).
The midpoint is calculated as follows
The vertical distance between (2,6) and (8,-6) is
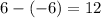
half of this is 6.
The horizontal distance between (2,6) and (8,-6) is
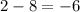
half of which is -3.
suntracting y = 6 and x = -3 to (2,6) gives
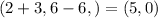
Hence, the coordinates of the centre are (a, b ) (5,0).
Now, the radius of the circle is half the distance to the midpoint
![\begin{gathered} r=\sqrt[]{6^2+(-3)^3} \\ r=3\sqrt[]{5} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/d5s7s8gi5b9vnw44ebl3qknvaom21fq4zn.png)
Hence, the equation for the circle is
![\begin{gathered} (x-5)^2+y^2=(3\sqrt[]{45}) \\ (x-5)^2+y^2=45 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/hdgj4bogru1oook9hhcx5eou84991n9j7x.png)