Question

Let f be the function defined by f(x) = cot(g(x)), where g is a differentiable function. Which of the following is equivalent to the derivative of f, with respect to x? A. −csc(g(x)) *g′(x)B. −csc²(g(x)) * g′(x)C. −csc(g′(x))D. −csc²(g(x))

Answer 1

To solve the present problem, we will be using some derivation properties such as chain rule

By the chain rule, we know that:

`(\partial F(G(x)))/(\partial x)=(\partial F(G(x)))/(\partial(G(x)))*(\partial G(x))/(\partial x)`

The derivative of G in respect to x, we can call it G'(x), because the G(x) is not given. From this, we are able to develop the given function derivative as follows:

`\begin{gathered} (\partial\cot(g(x)))/(\partial x)=(\partial\cot(g))/(\partial g)(\partial g(x))/(\partial x) \\ \\ (\partial\cot(g(x)))/(\partial x)=-\csc ^2(g(x))\cdot g^(\prime)(x) \end{gathered}`

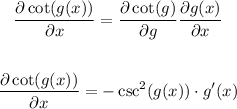
From the solution developed above we are able to conclude that the solution of the present question is:

B. - csc²(g(x))*g'(x)