Answer:
185 m
Explanation:
• The angle of depression from the water tower to a forest fire = 18°
,
• The height of the water tower = 60 meters
We want to find the horizontal distance on the ground between the water tower and the fire.
Let the distance = y
The diagram below illustrates the given information:
We then solve for y.
Using trigonometric ratios:
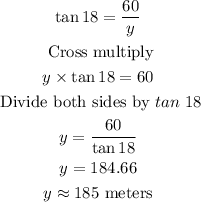
The horizontal distance on the ground between the water tower and the fire is 185 meters (rounded to the nearest meter).