Question

A cart that weighs 70 N is pushed 50 meters from rest with 1400 N. What is the finalvelocity of the cart?

Answer 1

Given data

*The given weight of the cart is W = 70 N

*The given distance is s =50 m

*The given initial velocity of the cart is u = 0 m/s

*The given force exerted on the cart is F = 1400 N

*The value of the acceleration due to gravity is g = 9.8 m/s^2

The mass of the cart is calculated as

`\begin{gathered} W=mg \\ m=(W)/(g) \\ =(70)/(9.8) \\ =7.14\text{ kg} \end{gathered}`

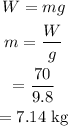
The formula for the acceleration of the car is given by the equation of motion as

`\begin{gathered} F=ma \\ a=(F)/(m) \end{gathered}`

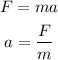
Substitute the known values in the above expression as

`\begin{gathered} a=(1400)/(7.14) \\ =196.0m/s^2 \end{gathered}`

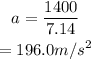
The formula for the final velocity of the cart is given by the equation of motion as

`v^2=u^2+2as`

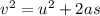
Substitute the known values in the above expression as

`\begin{gathered} v^2=(0)^2+2(196)(50) \\ v=\sqrt[]{19600} \\ =140\text{ m/s} \end{gathered}`

Hence, the final velocity of the cart is v = 140 m/s