Question

Which exponential relationships Below demonstrate a function that decays over time? Select all that apply. • y=20(1-0.23)^x • y=(2/3)^x • | x | y | | 0 | 1 | | 1 | 2 | | 2 | 4 | | 3 | 8 |

Answer 1

We are asked to determine which functions represent decay. A function represents a decay if the values of "y" decrease as the values of "x" increase. In more general terms, a function represents decay when the values of the dependent variables decrease as the values of the independent variable increase.

In the case of functions of the form:

`y=a(b)^x`

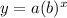
If we have:

`b<1`

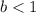
Then this function represents decay.

In the case of the function:

`y=20\mleft(1-0.23\mright)^x`

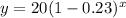
We have:

`b=1-0.23=0.77<1`

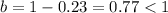
Therefore, this function represents decay

For the function:

`y=\mleft(2/3\mright)^x`

We have:

`b=(2)/(3)<1`

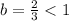
Therefore, this function represents decay.

In the case of the table we notice that the values of "y" increase as the values of "x" increase, therefore, this does not decay.