ANSWER
0.3764
Step-by-step explanation
Let X be the lifespan of the items. We know that it is normally distributed with a mean, μ, of 8.7 years and a standard deviation, σ, of 1.9 years,
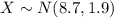
We have to find the probability that an item will last less than 8.1 years,
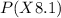
To find this, we have to standardize X as follows,
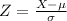
So we have,
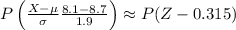
We have to check a z-score table to find this probability,
Note that we do not have sufficient accuracy in the table to find the exact value of z. But we do know that it is between -0.31 and -0.32, almost in the middle, so we can find a mean probability between these two,
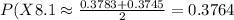
Hence, the probability that one item will last less than 8.1 years is, approximately, 0.3764.