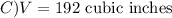
Step-by-step explanation
Step 1
The volume V of a given mass of gas varies directly as the temperature T and inversely as the pressure P
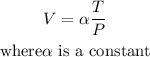
A measuring device is calibrated to give V = 124.8 in3 when T = 260° and P = 25 lb/in2 ,we can use this info to get the constant
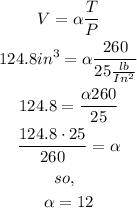
no, rewrite the equation
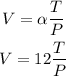
Step 2
What is the volume on this device when the temperature is 320° and the pressure is 20 lb/in2
replace

I hope this helps you