Question

1. Connor had $91.00 to invest. Part was invested at 16% and the rest at 14%. After one year, the total interest earned was $13.74. How much did he invest at each percent?answer QUICKLY please

Answer 1

Let x amount is invested at 16% rate, then remaining amount (91 - x) is invested in 14% rate.

Determine the intrest earned at 16% rate and at 14% rate after one year.

`\begin{gathered} I_(16)=(16)/(100)* x*1 \\ =(16x)/(100) \end{gathered}`

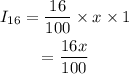
For 14% rate,

`\begin{gathered} I_(14)=(14)/(100)*(91-x)*1 \\ =(14(91-x))/(100) \end{gathered}`

The sum of the intrest is equal to 13.74. So,

`\begin{gathered} (16x)/(100)+(14(91-x))/(100)=13.74 \\ 16x+1274-14x=13.74\cdot100 \\ 2x=1374-1274 \\ x=(100)/(2) \\ =50 \end{gathered}`

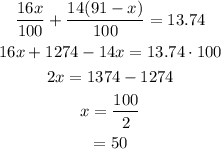
So $50 is invested at 16% rate.

Determine the invest amount in 14% rate.

`\begin{gathered} 91-x=91-50 \\ =41 \end{gathered}`

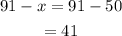
So invest amount in 14% rate is $41.

Thus answer is,

At rate of 16%: $50

At rate of 14%: $41