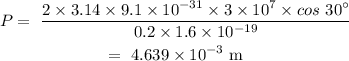Given:
The magnetic field is B = 0.2 T
The angle is

The speed of the helical path is
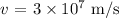
To find the pitch.
Step-by-step explanation:
The pitch can be calculated by the formula

Here, m is the mass of the electron whose value is 9.1 x 10^(-31) kg
q is the charge of the electron whose value is 1.6 x 10^(-19) C
On substituting the values, the pitch will be