Let T be the price of one taco, and B be the price of one burrito.
Since 3 tacos and 2 burritos cost $6.50, then:
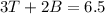
Since 3 tacos and 1 burrito cost $5.50, then:

Substract the second equation from the first equation. Left hand side minus left hand side equals right hand side minus right hand side:

Substitute B=1 into the second equation and isolate T to find the price of one taco:
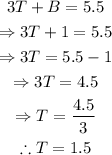
Therefore, the cost of one taco is $1.50.