The given problem can be exemplified in the following diagram:
In the diagram we have:

Now, the power supplied by the pushing force is given by:
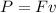
Where:
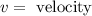
Therefore, we need to determine the value of the pushing force "F". To do that we will add the forces in the horizontal direction. We will take the forces to right as positive:
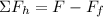
Since the object moves at a constant speed this means that the acceleration is zero and therefore, the sum of the forces is also zero, therefore:
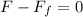
Now we solve for the pushing force by addig the friction force to both sides:
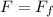
The friction force is given by:
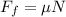
Where:
Now we need to determine the value of the normal force. To do that we will add the forces in the vertical direction. We will take the forces upwards as positive:
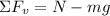
Since there is no movement in the vertical direction the sum of these forces must be zero, therefore:

Now we solve for the normal force by adding "mg" to both sides:
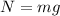
Now we substitute this value in the expression for the friction force:
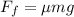
Now we substitute this value in the expression for the pushing force:
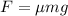
Now we substitute the given values:
![F=(0.7)(40\operatorname{kg})(9.8(m)/(s^2))]()
Now we solve the operations:
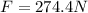
Now we substitute this value in the expression for power:

Solving the operations:
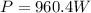
Therefore, the power supplied by the pushing force is 960.4 Watts.