Question

Find the equation of the line with Slope = −5-5 and passing through (3,−10)(3,-10) . Write your equation in the form y=mx+by=mx+b .

Answer 1

IDENTIFY AND SET UP

We are given the slope of a line and a point in which the same line passes through. We also know that the slope of a line can be obtained from the equation:

`\begin{gathered} s=(y-y_1)/(x-x_1) \\ \text{ where:} \\ (x_1,y_1)=\text{ point that line passed through.} \\ s=\text{slope} \end{gathered}`

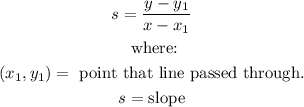
Our approach is to employ the formula above by substitute our coordinates.

EXECUTE

`\begin{gathered} -5=(y-(-10))/(x-3) \\ \text{ Cross multiplying, we get:} \\ -5x+15=y+10 \\ \text{Subtract 10 from both sides to get:} \\ y=-5x+15-10 \\ y=-5x+5 \end{gathered}`

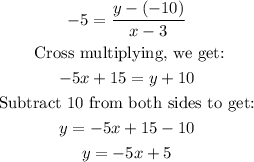
The equation of our line is y = -5x + 5