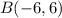Point M divides line segment AB in their midpoint.
This means that the segments AM and MB have the same length.
Be A(-4, 2) and M(-5,4) the coordinates of endpoint A and midpoint M:.
First step is to plot both poins and line segment AM:
To determine the coordinates of endpoint B, first you have to determine the distance between A and M with respect to their y-coordinates and x-coordinates.
Distance over the x-coordinates:
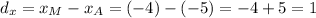
Next subtract this distance to the x-coordinate of M to calculate the x-coordinate of B:
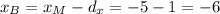
Distance over the y-axis:
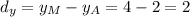
Next add it to the y-coordinate of M to calculate the y-coordinate of B:

The coordinates of B are: