mean of 65
standard deviation of 14
top 20% are selected
We need to use the Z-Score table to find the value of Z that represents 80%
In this case, the closest value would be 0.84 as can be seen in the picture below...
Now, we can use this equation...

where,
Z=0.84,
x is the cutoff score,
μ is the mean, and
σ is the standard deviation
Now, we replace those values and solve for x, as it follows,
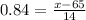

Therefore, the cutoff score is 76.76