Answer:
397 shelter openings are needed
Explanations:
This is a normal distribution
Mean = 250
Standard Deviation = 75
There are not enough beds 95% of the time
Confidence Level = 95/100 = 0.95
Significance Level (SL) = 1 - Confidence Level (CL)
SL = 1 - 0.95
SL = 0.05
Z-score at 0.05 Significance Level = 1.96
z = 1.96

Let the number of shelter openings be represented by Y
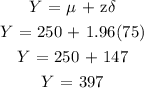
397 shelter openings will be needed