Step-by-step explanation
The value of number e is 2.71828... with infinite decimals. We can use different expressions to approximate it. For example let's analyze the end behaviour of the expression (1+1/n)^n i.e. its limit when n tends to infinite. In order to do this we can calculate its value for very large values of n:
![\begin{gathered} n=1000\Rightarrow(1+(1)/(1000))^(1000)=2.71692... \\ n=10000\Rightarrow(1+(1)/(10000))^(10000)=2.71814... \\ n=100000\operatorname{\Rightarrow}(1+(1)/(100000))^(100000)=2.71826... \end{gathered}]()
As you can see the greater the value of n the closer the expression is to e.
√5 is equal to 2.23606... which is further from e than the limit of the expression above and C/d is a rational number whereas e is irrational so we can conclude that the expression of the third option is better to estimate e than those of the first two options.
We can compare it to the expression of the last option (1+n)^(1/n) by analyzing its end behaviour:
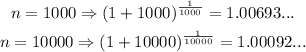
As you can see this expression doesn't approach to e when n tends to infinite.
Answer
Then the answer is the third option.