ANSWER
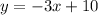
Step-by-step explanation
The general form of the equation of a line is:

where m = slope
b = y-intercept
A line that is perpendicular to another line has a slope that is the negative inverse of the slope of the line.
Hence, the slope of the line we are looking for is:
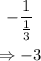
Now, we can apply the point-slope method to find the equation of the line:

where (x1, y1) = point that the line passes through
Therefore, the equation of the line is:
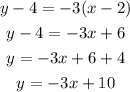
That is the answer.