Given the inequality:
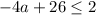
You can solve for "a" as follows:
1. Apply the Subtraction Property of Inequality by subtracting 26 from both sides of the inequality:
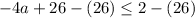
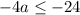
2. Apply the Division Property of Inequality by dividing both sides by -4. As you are dividing both sides by a negative number, the direction of the inequality symbol changes:
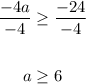
Hence, the answer is: Second option.