Question

Solve for x if the equation is.x^2/x+4 = 16/x+4

Answer 1

To solve the equation:

`(x^2)/(x+4)=(16)/(x+4)`

we multiply both sides by (x+4), then we get:

`(x^2)/(x+4)\cdot(x+4)=(16)/(x+4)\cdot(x+4)`

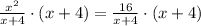
then we get:

`\begin{gathered} x^2=16 \\ x=\pm\sqrt[]{16} \\ x=\pm4 \end{gathered}`

Now, from this we may conclude that the equation has two solutions, x=4 and x=-4. But we need to be careful with this, from the orginal expression we notice that the denominator in both sides is (x+4), then if we plug x=-4, the result in both denominators will be zero but we know that the division by zero is not defined.

Taking the above into account we conclude that the solution for the equation is x=4.