Question

2) How many liters of N2 gas at 1.03 atm and 22.36 C are produced by the decomposition of 160.7 g of NaN3?Keep the answer with 2 decimal places.2NaN3(s) --> 2Na(s) + 3N2(g)

Answer 1

87.24 L.

Step-by-step explanation:

What is given?

Pressure = 1.03 atm.

Temperature = 22.36 °C + 273 = 295.36 K.

R = 0082 L *atm/mol*K.

Mass of NaN3 = 160.7 g.

Molar mass of NaN3 = 65 g/mol.

Step-by-step solution:

We have to find the number of moles of N2 to use the ideal gas law which has the formula:

`PV=nRT.`

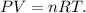
Where P is pressre, V is volume, n is the number ofmoles, R is the idelal gas constant and T is tthe hemperature on the Kelvin scale.

First, we have to convert 160.7 g of NaN3 to moles using its given molar mass, like this:

`160.7\text{ g NaN}_3\cdot\frac{1\text{ mol NaN}_3}{65\text{ g NaN}_3}=2.47\text{ moles NaN}_3.`

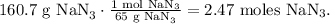
In the chemical equation, you can see that 2 moles of NaN3 reacted produces 3 moles of N2, so by doing a rule of three, we're going to find how many moles of N2 are being produced by 2.47 moles of NaN3:

`2.47\text{ moles NaN}_3\cdot\frac{3\text{ moles N}_2}{2\text{ moles NaN}_3}=3.71\text{ moles N}_2.`

Now that we have the number of moles of N2, we can solve for 'V' in the initial formula and replace the given values that we have, like this:

`V=(nRT)/(P)=\frac{3.71\text{ moles}\cdot0.082(L\cdot atm)/(mol\cdot K)\cdot295.36K}{1.03\text{ atm}}=87.24\text{ L.}`

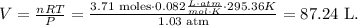
The answer i that hthe volume in liters of N2 gas is 87.24 L.