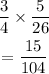The arithmetic problem shown is:
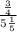
First of all, we have to convert the mixed number in the denominator to an improper fraction. Let's do it:
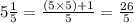
Now, the arithmetic becomes:
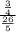
Remember, when we are dividing by a fraction, we are actually multiplying by its reciprocal. Thus, we do:
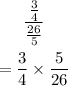
Now we simply multiply straight and reduce (if possible). Shown below: