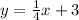Question

Write an equation for a line perpendicular to 3y+12x=-15 and passing through the point (-4,2) Answer must be written as y=

Answer 1

The product of the slopes of the perpendicular lines is -1

That means if the slope of one of them is m, then the slope of the other is -1/m

We reciprocal the value and change the sign

In the form of the equation

`ax+by=c`

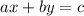
The rule of the slope is

`m=-(a)/(b)`

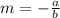
Since the given equation is

`3y+12x=-15`

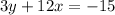
Then a = 12 and b = 3

Use them in the rule above to find the slope

`\begin{gathered} m=(-12)/(3) \\ m=-4 \end{gathered}`

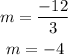
The slope of the given line is -4

To find the slope of the perpendicular line:

Reciprocal 4 and change the sign from negative to positive

The slope of the perpendicular line is

`m_p=(1)/(4)`

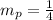
Since the form of the linear equation is

`y=mx+b`

m is the slope

b is the y-intercept

Since the slope of the line is 1/4, then

`y=(1)/(4)x+b`

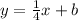
To find b use the given point (-4, 2)

Substitute x in the equation by -4 and y by 2

`\begin{gathered} 2=(1)/(4)(-4)+b \\ 2=-1+b \end{gathered}`

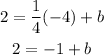
Add 1 to both sides

`\begin{gathered} 2+1=-1+1+b \\ 3=b \end{gathered}`

The equation of the perpendicular line is

`y=(1)/(4)x+3`