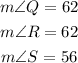Given the triangle QRS, you know it is isosceles with base QR, and:
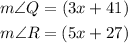
By definition, an Isosceles Triangle is a triangle that has two equal sides and two equal angles. Therefore, you can determine that, in this case:
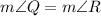
Then, you can set up the following equation with the expressions that represent each angle:
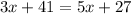
Solve for "x" in order to find its value:
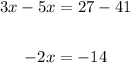
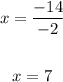
Knowing the value of "x", you can determine that:
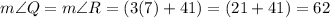
By definition, the sum of the interior angles of a triangle is 180 degrees. Therefore, you can set up this equation:
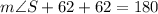
Solving for angle S, you get:

Hence, the answer is: